Mapping the magnetic field of an object is simple in principle. First, measure the strength and direction of the magnetic field at a large number of locations (or at every point in space). Then, mark each location with an arrow (called a vector) pointing in the direction of the local magnetic field with its magnitude proportional to the strength of the magnetic field.

An alternative method to map the magnetic field is to 'connect' the arrows to form magnetic field lines. The direction of the magnetic field at any point is parallel to the direction of nearby field lines, and the local density of field lines can be made proportional to its strength.

Magnetic field lines are like the contour lines (constant altitude) on a topographic map in that they represent something continuous, and a different mapping scale would show more or fewer lines. An advantage of using magnetic field lines as a representation is that many laws of magnetism (and electromagnetism) can be stated completely and concisely using simple concepts such as the 'number' of field lines through a surface. These concepts can be quickly 'translated' to their mathematical form. For example, the number of field lines through a given surface is the surface integral of the magnetic field.

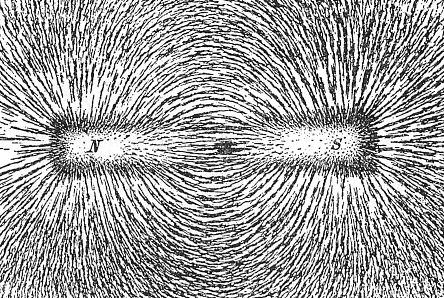
Various phenomena have the effect of "displaying" magnetic field lines as though the field lines are physical phenomena. For example, iron filings placed in a magnetic field line up to form lines that correspond to 'field lines'. Magnetic fields' "lines" are also visually displayed in polar auroras, in which plasma particle dipole interactions create visible streaks of light that line up with the local direction of Earth's magnetic field.

Field lines can be used as a qualitative tool to visualize magnetic forces. In ferromagnetic substances like iron and in plasmas, magnetic forces can be understood by imagining that the field lines exert a tension, (like a rubber band) along their length, and a pressure perpendicular to their length on neighboring field lines. 'Unlike' poles of magnets attract because they are linked by many field lines; 'like' poles repel because their field lines do not meet, but run parallel, pushing on each other. The rigorous form of this concept is the electromagnetic stress–energy tensor.